A paradox is a statement that leads to an unacceptable or contradictory result even when the reasoning seems logical and correct. While some paradoxes are invalid in a practical sense, they have been used to promote critical thinking. Some help reveal errors in definitions or theories held to be absolute in philosophy, sciences, and even theology. For example, the Olbers’ Paradox helped prove wrong the previously held belief that the universe is both infinitely big and infinitely old, and not expanding. If that were true, the entire night sky would have to be as bright as the Sun’s surface. So, here is a list of some amazing paradoxes that are sure to make your head spin.

1 Ant on a Rubber Rope

An ant crawling on a rubber rope can reach the end even if the rope stretches faster than the ant’s crawl.

The Ant on a Rubber Rope paradox is a mathematical puzzle with a counterintuitive solution. The puzzle asks,  “If an ant is crawling along a one-kilometer long, taut, rubber rope at one centimeter-per-second speed. If the rope stretches at a constant speed of one kilometer per second, would the ant ever be able to reach the end?”

Though it may seem impossible, the answer is “yes,” because as the rope stretches, it also moves the ant forward. So, the ant can reach the end of the rope, though it would take a very long time. However, if the rope stretches with an increasing speed, then the ant won’t be able to reach the end. A similar paradox is the Achilles and the Tortoise paradox in which Achilles gives the tortoise a head start but always finds himself running to cover the distance the tortoise had already moved.

The puzzle can be applied to the question of whether light from galaxies extremely far away would ever reach Earth considering the universe is expanding. If the expansion had been at a constant rate, then just like the ant, the light could reach us. However, since the expansion is accelerating, it might not. (source)

2 Coastline Paradox

As the unit of measurement grows smaller the length of a coastline increases to infinity.

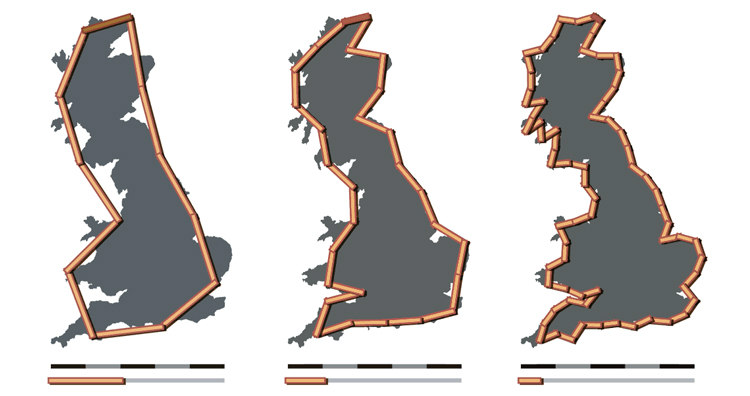
The Coastline Paradox is a counter-intuitive observation about how coastlines do not have a well-defined length. The phenomenon was first observed by Lewis Fry Richardson who was searching for a relationship between the probability of two countries going to war and the length of their common border. As he collected data, he found that there is a considerable difference between various published lengths of the international borders. Following the observation, Richardson demonstrated that the measured length of coastlines, or any other natural features, increases limitlessly as the unit of measurement becomes smaller.

Depending on the method used and how much cartographic generalization has been done, the measured length of the coastline changes. This is because of the fractal-like properties of coastlines. As any landmass has detail no matter how closely you look at it, at kilometer scale or at microscopic scale, there is no well-defined perimeter to measure the landmass. (source)

3  Grelling–Nelson Paradox

This paradox concerns the applicability of a word to itself. The word “heterological” means “inapplicable to itself.” So, is “heterological” a heterological word?

The Grelling–Nelson Paradox is an antinomy, referring to a real or apparent mutual incompatibility or a self-contradiction. It was proposed by Kurt Grelling and Leonard Nelson in 1908. If the answer to the above question is no, then the word “heterological” does describe itself which would mean the word “heterological” is heterological, making the answer a contradiction. If the answer is yes, then “heterological” does not describe itself, but that would mean “heterological” is not a heterological word, which again is a contradiction.

A variation of the Grelling-Nelson Paradox is the Barber Paradox. There is a barber who shaves all those, and only those, who do not shave themselves. So, does the barber shave himself? Answering the paradox either way leads to a contradiction. The paradox is based on Russell’s Paradox or Russell’s Antinomy formulated by British mathematician Bertrand Russell to show that the formalizations of naïve set theory by George Canto led to contradictions. (source)

4 Ship of Theseus

If you had a boat whose decaying planks you replaced with new ones over the course of time so that all the planks are now replaced, would it be the same boat?

Theseus’s Paradox is a thought experiment that questions the idea of identity and appears in several practical fields. In law, for example, the paradox is often encountered in cases of ownership rights and battles. Sports teams, musical bands, or companies may see their old members become rivals which results in the need for legal actions between the old and new groups.

A continuation of the paradox states that if all the decaying planks were stored in a warehouse, and in time a new technology enabled you to restore the planks to normal, would the new ship you build with those planks be the original ship? If so, what will that make the other ship you repaired by replacing decaying planks? A real-life example of this paradox is the British girl group Sugababes. All the original members left the band to form a different band while the old band kept replacing members and operating under the original name. (source)

5 False Positive Paradox

This is a situation in which a highly accurate test fails if the test conditions are rare enough. For example, if 10 people in a population of 10 million are infected with a disease, and the test to identify them is 99% accurate, then 99.9999% of positives will be false.

The False Positive Paradox is a statistical result which has more numbers of false positives than true positives when the overall population has a low incidence of the disease or a condition even when the test has proven accurate when used on a high-incidence population. It is what is known as the “veridical paradox,” one that seems absurd but can be demonstrated to be true.

According to above example, if the test is 99% accurate, that means it is one percent inaccurate and 100,000 people will receive positives, among which, only 10 are true positives and 99,990 are false positives making the test 99.99% inaccurate. The counter-intuitive results of the False Positive Paradox occur because the test wasn’t modified to account for the low incidence of the disease in the selected population. (source)

6 Omnipotence Paradox

Could God create a stone so heavy that even He could not lift it?

The Omnipotence Paradox arises from the understanding of the term “omnipotent being” as someone with no limits and capable of bringing about any outcome including illogical and contradictory ones. The paradox is a source for debate between theologians and atheists. While some theologians reject the no-limits understanding of omnipotence or believe that omnipotence doesn’t mean breaking the laws of logic or nature, some argue that the paradox is meaningless and the phrase “could not lift” doesn’t make sense because God is omnipotent.

Other possible resolutions ask for re-examining the definition of omnipotence and how it is applied to God, or if the definition is applied towards God himself or towards his external surroundings. There are other alternative statements of the paradox such as, “If given the axioms of Riemannian geometry, can an omnipotent being create a triangle whose angles do not add up to 180 degrees?” and “Can God create a prison so secure that he cannot escape from it?”. (source)

7 Potato Paradox

If you have 100 pounds of potatoes which are 99% water by weight and you let them dry so that they are 98% water, their new weight is 50 pounds.

The potato paradox is a mathematical calculation that results in a counter-intuitive answer. There are several mathematical proofs for the paradox. If the potatoes are 99% water, that would mean the solids weigh one pound which will not change even when they are dehydrated. Say, the new weight of the potatoes is x pounds. So,

x = 1 + (98/100)x

which when solved gives,

x = 50 pounds

Another way to prove it is through ratios. In the beginning, the ratio of solids-to-water is 1:99. After they are dehydrated, the water decreases to 98%, which would mean the solids account for 2% of the weight. The new ratio is 2:98, or 1:49. So, the water’s weight is 49 pounds and the dehydrated potatoes weigh 50 pounds.

The answer will remain the same as long as the percentage of the solid part becomes doubled. So, if the potatoes have 99.9% water, dehydrating so that there is 99.8% would still halve the total weight. The same is true for 99.99% of water initially and 99.98% after dehydration, and so on. (source)

8  Simpson’s Paradox

This is a paradox in probability and statistics where a trend seen in different groups of data disappears or reverses when the groups are combined: In 1995 and 1996 baseball games, David Justice had a higher batting average than Derek Jeter. But, when the two years are combined, Jeter’s average is higher.

Simpson’s Paradox, or the Yule–Simpson effect, was first described by Edward H. Simpson in 1951 and a similar effect was described by statisticians Karl Pearson et al., in 1899 and Udny Yule, in 1903. Often encountered in social-science and medical-science statistics, it is quite problematic when data is interpreted casually. It was also used to explain to laymen or non-specialists about the kind of misleading results wrongly applied statistics can yield.

Another well-known example of the paradox is a study done in 1973 on gender bias in graduate school admissions at the University of California, Berkeley. The study showed that on the whole, men were significantly more likely to be admitted than women. But, when examining individual departments, there was either no bias or a small but statistically significant bias in favor of women. This was because, according to the research done by Bickel et al., women applied to competitive departments which are difficult to get in, while men applied to less competitive departments that are easy to get in. (source)

9 Crocodile Paradox

A crocodile, who has kidnapped a child, promises the parent that the child would be returned if and only if they correctly predict what the crocodile will do next.

The Crocodile Paradox belongs to the family of paradoxes known as “liar paradoxes” which try to show that the common beliefs we have about truth and fallacy can lead to contradictions. In the case the parent guesses that the crocodile will return the child, if the crocodile had decided not to, then the child will not be returned and if he decided otherwise, then the child will be returned.

But, in the case the parent guesses that the child will not be returned, if the crocodile decided to keep the child he will violate his terms, and if he decided to return the child he would still violate the terms because that would mean the parent’s guess was wrong and so the child shouldn’t be returned.

It is as good as impossible to find the right solution and the question of what the crocodile should do is a paradox. Another example of a liar paradox is the Pinocchio Paradox. What will happen if Pinocchio says “My nose will grow now”? (source)

10 The Bootstrap Paradox

A time traveler copies a mathematical proof from a textbook and travels back in time to meet the mathematician who first published it before the day it was published. But the mathematician simply copies the proof and publishes it as his own. So, where did the proof originally come from?

Bootstrap Paradox, also known as a “causal loop,” is a sequence of events in which an event results in another event, which in turn was the cause of the first event. The term “bootstrap” refers to the phrase “pulling yourself up by your bootstraps,” and to the time travel story By His Bootstraps by Robert A. Heinlein. A causal loop is a popular theme among many science fiction stories, especially those involving space travels.

A similar example is described by the doctor in Doctor Who. A fan of Beethoven takes the music sheets back in time to get an autograph and finds out there never was anyone named Beethoven. So, he publishes the music in Beethoven’s name instead. The question is, where did the music originally come from?

In the Star Trek franchise, the term “predestination paradox” is used to refer to “a time loop in which a time traveler who has gone into the past causes an event that ultimately causes the original future version of the person to go back into the past.” Another usage of the term predestination paradox is to refer to specific situations in which a time traveler goes back in time to prevent an event, but ends up causing the event. The film Predestination is based on this definition. (source)